High-order Adaptive Method for Computing Two-dimensional Invariant Manifolds of Maps

Jacek Wróbel and Roy Goodman. Communications in Nonlinear Science and Numerical Simulation, 2013.
Jan 1, 2013·
Jacek Wróbel
Jacek Wróbel
Roy Goodman
Roy Goodman
· 1 min read
Abstract
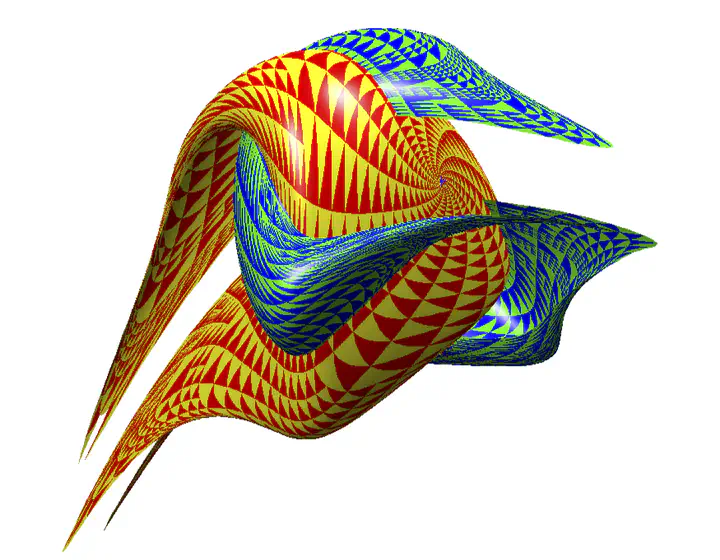
An efficient and accurate numerical method is presented for computing invariant manifolds of maps which arise in the study of dynamical systems. A quasi-interpolation method due to Hering-Bertram et al. is used to decrease the number of points needed to compute a portion of the manifold. Bézier triangular patches are used in this construction, together with adaptivity conditions based on properties of these patches. Several numerical tests are performed, which show the method to compare favorably with previous approaches.
Type
Publication
Communications in Nonlinear Science and Numerical Simulation, 18(7), 1734–1745
publication published

Part 2 of Jacek Wróbel’s dissertation.

Jacek Wróbel
Authors
Jacek Wróbel
Member of Technical Staff, Draper Laboratories

Jacek is a simulation engineer at Draper Labs in Cambridge, MA.

After completing his Ph.D. under the supervision of Roy Goodman, Jacek spent a year as a postdoc at NJIT working with Michael Booty and Michael Siegel. He spent three years as a postdoc at Tulane University with Lisa Fauci and Ricardo Cortez. He worked at Raytheon before beginning his current job as member of technical staff at Draper Laboratories.

Roy Goodman
Authors
Roy Goodman
Professor, Associate Chair for Graduate Studies, Department of Mathematical Sciences

I am a professor in the Department of Mathematical Sciences at NJIT. My research applies methods of dynamical systems to (conservative, dispersive) nonlinear wave equations arising, for example, in optics and fluid mechanics. I am interested in dynamical and bifurcation phenomena, and I use techniques from Hamiltonian mechanics and perturbation theory, as well as extensive computations. My current research in vortex dynamics is supported by the NSF under DMS-2206016.

I led the effort to start the SIAM New York-New Jersey-Pennsylvania Section (SIAM NNP) and was its first president in 2023-2024. I have handed over the reins to a new leadership group.